This article describes why the power in pure inductive and capacitive circuits is zero. The inductors and capacitors are the basic building blocks of an electric circuit, and you will understand the concept of no power drawn by these elements after reading this article. Let’s dive into the details.
Table of Contents
Power in a Pure Inductive Circuit
The active power drawn by a pure inductive and a capacitive circuit is zero. In a pure inductive circuit, the current lags the voltage by 90° because the inductive load always opposes the rate of change of current.
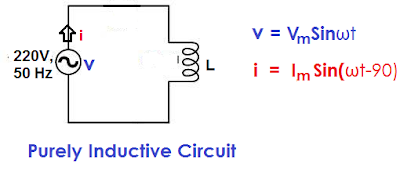
The back EMF or counter EMF is generated in the inductive load due to the rate of change of the current. The back EMF generated in the inductive circuit is given by.
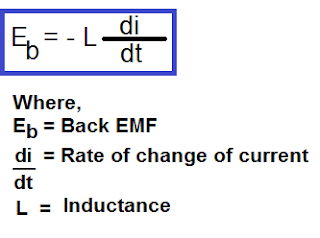
The minus sign indicates that the voltage induced in the inductive circuit opposes the applied voltage. Because of this opposition, the current through the inductive load lags the voltage. In a purely inductive circuit, the current lags the voltage by 90 degrees. The waveform of current and voltage in the purely inductive circuit is shown below.
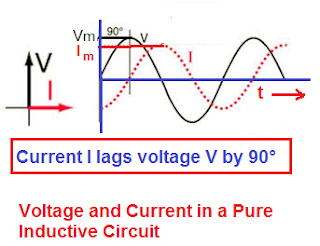
The voltage and current in a purely inductive circuit do not rise or fall together. There is always a phase shift of 90° between voltage and current. The power factor of the purely inductive circuit is ;
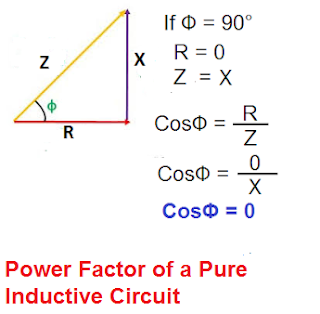
The power in an AC circuit is given by;
P = VICosΦ ———–(1)
The power factor of the purely inductive circuit is zero(lagging).
CosΦ = 0 ———–(2)
The power in a purely inductive circuit is given by,
P = VICosΦ
P = VI x 0
P = 0
Thus, the pure inductive circuit consumes zero active power, and it consumes reactive power only from the supply source.
Power in a Pure Capacitive Circuit
In a purely capacitive circuit, the current leads the voltage by 90° because the capacitive load always opposes the rate of change of voltage. The Pure capacitive circuit is given below.
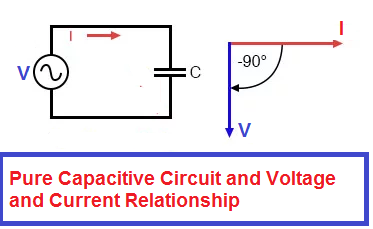
The current through the capacitor leads the applied voltage by 90°in a purely capacitive circuit.

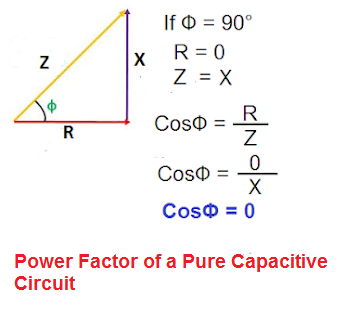
The Power factor of a pure capacitive load is zero(leading).
The power in an AC circuit is given by;
P = VICosΦ ———–(3)
The power factor of the purely capacitive circuit is zero(leading).
CosΦ = 0 ———–(4)
The power in a purely inductive circuit is given by,
P = VICosΦ
P = VI x 0
P = 0
Thus, a pure capacitive circuit consumes zero active power. The pure capacitive circuit consumes reactive power from the supply source.
Mathematical Proof:
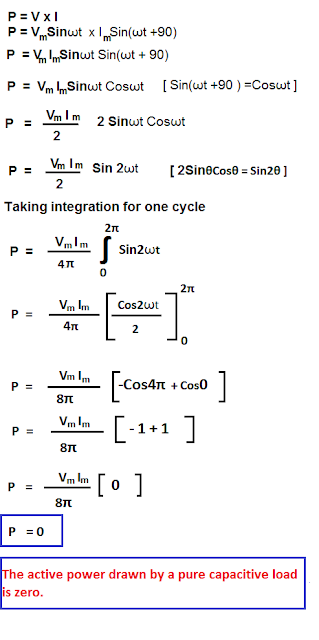
The active power drawn by a pure capacitive load can be mathematically proved to be zero.
I really appreciate thanks for your guidance.