The transformer is rated in KVA, not in KW. It supplies power to electrical loads like motors and heaters, which have their own power factor.

The power factor at the transformer’s secondary and primary is equal to the combined power factor of all electrical loads connected to the transformer. Thus, the transformer does not have a fixed power factor, and the power factor varies with the types of load connected to it and also on the percentage loading on the transformer.
The transformer is a link between the alternator and the load. The alternator supplies active and reactive power to the load. Similarly, the transformer is a power supply source that supplies the active kW and reactive kVAR to electrical loads. The volts x Amps equation of the transformer gives the maximum current that the winding can carry. The transformer winding can’t carry current more than its rated current carrying capacity. The same kVA and more MVA mean winding can carry more current without overheating.
If the transformer is rated in kW, the designer has to fix the kVAR capacity of the transformer. However, kVAR depends on the types of loads connected to the transformer, and it is not possible to fix the kVAR delivering capacity of the transformer.
In other words, if the transformer is rated based on KW, the user has to operate the transformer at the designed power factor of the transformer. However, it is not pragmatic to operate the transformer at a particular power factor or a particular kVAr because the load power factor varies, and the system power factor changes with variation in the power factor of the connected loads.
The transformer designer does not know at what power factor the customer will operate the transformer. The designer gives the choice to operate the transformer at whatever power factor by rating the transformer in apparent power (KVA or MVA).
The apparent power(kVA) of the transformer is equal to the vector sum of active power(kW) and reactive power(kVAr).
kVA⃗= kW⃗+ kVAr⃗
kVA2= kW2 + kVAr2
kVA =√(kW2 +kVAr2) ——–(1)
Where,
kVA – Apparent Power
kW – Active Power
kVAr – Reactive Power
From equation(1), if the load is resistive, then the power factor is unity, and kVAr =0
kVA = kW ( if the load is resistive)
If the power factor is low, the kVAR supplied by the transformer will increase, and correspondingly, the active power delivery will decrease. The transformer will be underutilized if the power factor of the loads is low.
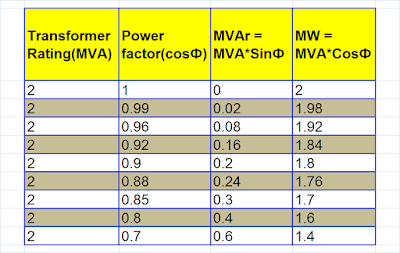
From the above table, it is clear that the active power delivering capacity of the transformer gets lowered with a lower power factor.
To fully utilize the transformer, the power factor should be high. The better the power factor, the lesser the kVAr demands by the electrical system, and thus, the transformer’s active power-delivering capacity can be increased.
The power factor can be improved by installing capacitor banks to neutralize the reactive kVAr with capacitive kVAr.
HOW CAN I GET THE PDF ON THIS TOPIC
Very good knowledge sir
Aap ke es matter se bahut knowledge mila
Very very thanks